Parametric Equations

In my last couple of months I have been fascinated by parametric curves. They are incredibly beautiful. So, I decided to show you some of my favorite ones and also a small introduction about them.

Firstly, a parametric equation of a curve express the coordinates of the points of the curve as functions of a variable, called a parametric.Most known are x = sin t  and y = cos t , which are parametric equations for the unit circle, where t is the parameter. Together, these equations are called a parametric representation of the curve.

The examples that everyone knows when it come to graphs (parabola, circle, ellipse, parabola and so on) all can be represented as parametric equations. The ones that I find more interesting are the ones that have this representation: x = f(sin t, cos t) and y = g(sin t, cos t) ( the coordinates x and y are functions of sin t and cos t). The unit circle is the basic one, but the ellipse can also be written like this:

An ellipse in general position can be expressed as

as the parameter t varies from 0 to 2π. Here is the center of the ellipse, and is the angle between the -axis and the major axis of the ellipse.

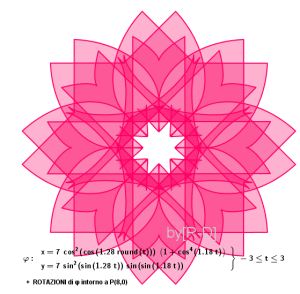
And now comes the funny part… The more complicated ones. So here are some my favorite examples:

 

You can see that all my examples use just functions in sin and cos. Also, the colors used make them more nicely (but creativity is ‘at home’ everywhere). You can check my Facebook album if you want to see more and also my Pinterest album . Right now I am more into the 2D shapes, but for sure 3D ones will become of interest very soon.

If you are interested in these things you can trying drawing some of your ones here (this website gives you a good feeling how you can construct them). As always the Wikipedia page is useful (and I have used it as reference for my examples and definitions). Moreover Khan Academy has a couple of interesting videos about parametric equations, their use in other fields (physics) and also about representing some of them.

I haven’t tried writing this type of articles before, but let me know if you would like to see more like this one. (I could try and write small articles about different math concepts; this one was fun and constructive to write.) Any feedback is appreciated so use the like and share buttons!

Let me know what you think about this series. Enjoy the day. If you have ideas for future blog posts, let us know.  You can find us on  Facebook, Tumblr, Google+, Twitter and  Instagram. We will try to post there as often as possible.

Lots of love and don’t forget that maths is everywhere! Enjoy!